The line surface, to which there exists a unique tangent plane in any line located on the surface,
while the surface is located in the neighbourhood of this line
in one halfspace determined by the tagent plane ,
is the developable surface.
DEVELOPABLE SURFACES - TORSES

The line surface, to which there exists a unique tangent plane in any line located on the surface,
while the surface is located in the neighbourhood of this line
in one halfspace determined by the tagent plane ,
is the developable surface.
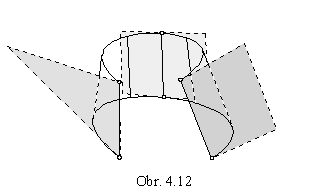
All points located on the developable surface are parabolic points (Fig. 4. 12).
Developable surfaces are:
I. Special types of developable surfaces
1. Cylindrical surface
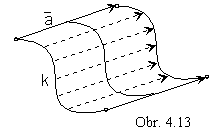
Translating the curve segment k in the direction of the non-zero translation vector a cylindrical surface can be created
(Fig. 4. 13).
 Synthetic representation:
Synthetic representation:
Analytic representations:
basic figure - r(u)=(x(u), y(u), z(u), 1), uÎ<0,1>
generating principle - class of translations determined by the vector
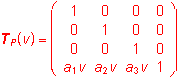 , vÎ<0,1>
, vÎ<0,1>
modelled figure - p(u,v)=r(u).TP(v)=(x(u)+a1v, y(u)+a2v, z(u)+a3v, 1), (u,v)Î<0,1>2
If the basic curve segment k is a plane segment, k
![]() a and vector a is the direction vector of the plane a,
a and vector a is the direction vector of the plane a,
the created surface patch is the part of the plane a (Fig. 4. 14).
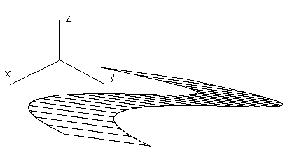
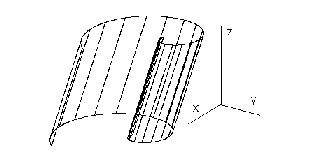
Fig. 4. 14 Fig. 4. 15
 If the basic curve segment k is the circle in the plane a
If the basic curve segment k is the circle in the plane a
and vector a is not the direction vector of the plane a, a x a,
the patch of the cylindrical surface can be created,
for the direction vector a^a it is a patch of the cylindrical surface of revolution.
Illustration of the cylindrical surface patch with the basic curve in the Archimedean spiral is in the Fig. 4. 15.
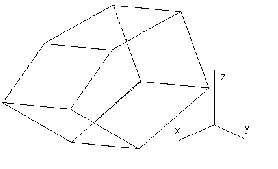
If the basic curve segment is the polygon
and vector a is not parallel to any of the polygon sides,
modelled surface patch is the part of the prismatic surface
Fig. 4. 16
with the edges in the direction of vector a (surface created by planar patches).
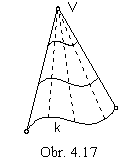
2. Conical surface
From the basic curve segment k a conical surface patch can be created by scalings to the given real point- centre V
with a non-zero coefficient h (Fig. 4. 17).
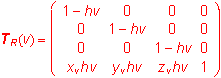 , vÎ<0, 1>
, vÎ<0, 1>
modelled figure -
p(u, v)=r(u).TR(v)=((1-hv) x(u)+xv hv, (1-hv) y(u)+yv hv, (1-hv) z(u)+zv hv,1), (u, v)Î<0,1>2
Non-zero coefficient hof the scalings determines the surface patch form:
Fig. 4. 18
If the basic curve segment k is a plane segment in the plane a ans in this plane also vertex V is located,
the created surface patch is a part of the plane a (Fig. 4. 18, coefficient h<1).
If the basic curve segment k is a circle in the plane a and centre V is not located in the plane a,
the created surface patch is the circular conical surface patch with the vertex V.
If the line passing through the point V perpendicularly to the plane a
intersects this plane in the centres of the basic circle k,
the created conical surface patch is the surface of revolution.
Illustration of the conical surface patch
with the basic curve in the Folium of Descartes is in the Fig. 4.19, coefficient h>1.
If the basic curve segment is the polygon k and centre V is not the point of this line,
modelled surface patch is the pyramidal surface patch with the vertex in the point V
(surface composed from planar patches) (Fig. 4. 20, coefficient h=1).
Fig. 4. 19 Fig. 4. 20
3. Surface of tangents to a curve
Translating points on basic curve segment in the direction of tangent vectors to the basic curve segment in these points the surface of tangent lines to a curve can be created( Fig. 4. 21).

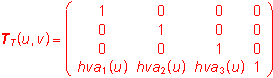 , (u,v)Î<0,1>2, h
, (u,v)Î<0,1>2, h
modelled figure - p(u, v)=r(u).TT(u,v)= (x(u)+hva1(u), y(u)+hva2(u), z(u)+hva3(u), 1), (u, v)Î<0,1>2
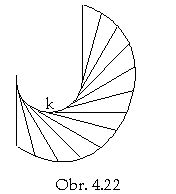
If the basic curve segment k is a planar segment, k
![]() a,
a,
tanget vector r´(u) at any point on the curve segment
is the direction vector of the plane a
and the modelled surface patch is a part of the plane a (Fig. 4. 22).
For the space basic curve segment
tangent vectors are non-collinear and non-complanar vectors (Fig. 4. 23).
If the basic curve segment is a helix,
the only one developable helical surface can be created,
surface of tangent lines to the helix (Fig. 4. 24).
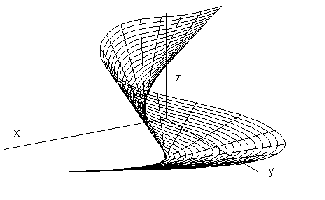

Fig.4. 23 Fig. 4. 24
4. General developable line surface
The line surface can be determined by two basic curves (u-parametric curves on the surface),
while any line located on the surface (v-parametric curve)
is passing through one point on each of the basic curves.
For the suitably chosen pairs of corresponding points on basic curves only,
(suitable parametrisation of point functions, which are analytic representatins of basic curves)
we can create the developable surface.
Generating principle in the synthetic representation of the general developable surface
is a linear interpolation (Fig.4. 25).
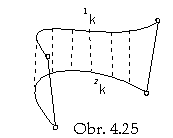
Cylindrical surface can be created from two congruent basic curves 1k and 2k, while one of them was created by the translation of the other one. Translation vector determines the direction of lines on the cylindrical surface.
For conical surface and surface of tangent lines to a curve are these two curves 1k and 2k homothetical (Fig. 4. 26),
or one of them is represented by a constant point function, it is a point - vertex of the conical surface.
Transition surface patch (type e.) determined by an ellipse and a closed polygon is in the Fig. 4. 27, it is created by four different triangles (parts of surface tangent planes) and four different conical patches with vertices in the vertices of the basic polygon and boundary line segments in the sides of the separate triangles.
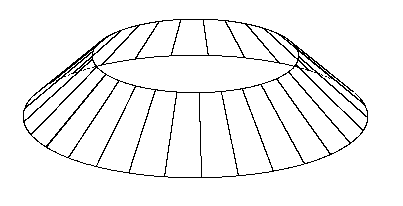

Fig. 4. 26 Fig. 4. 27
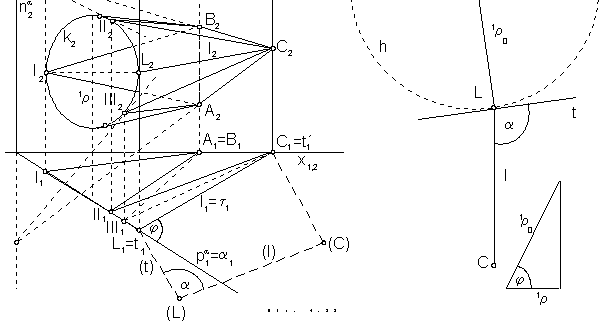
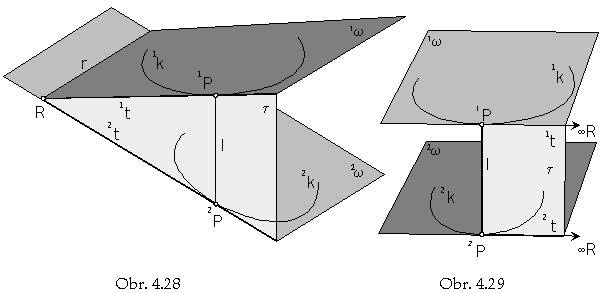
Line on the developable surface can be determined by the tangent plane to the surface in this line.
Curve 1k has in the point 1P osculating plane 1w, in which the tangent line 1t to the curve in the point 1P is located.
Tangent line 1t is also located in the tangent plane t to the surface
(t is the set of tangent lines to all curves located on the surface in the given point).
Point 2P on the curve 2k , which determines with the point 1P the line l=1P 2P on the surface
can be constructed as the tangent point on the tangent line 2t to the curve 2k,
located in the osculating plane 2w and also in the tangent plane t.
Lines 1t, 2t are intersection lines of the plane t and osculating planes 1w, 2w
and they can have only a non-empty intersection, common real or ideal point.
Their superposition determines the position of osculating planes 1w, 2w.
1w Ç 2w = r 1t Ç 2t = R, RÎr (Fig. 4. 28)
1w Ç 2w =
![]() r 1t Ç 2t =
r 1t Ç 2t =
 R (Fig. 4. 29)
R (Fig. 4. 29)
II. Developement of the surface
Developable line surface is the envelope of the space between two basic curves 1k, 2k created by tangent planes.
Development of the surface is the isometric mapping of the surface to the plane, for which the following rules are valid:

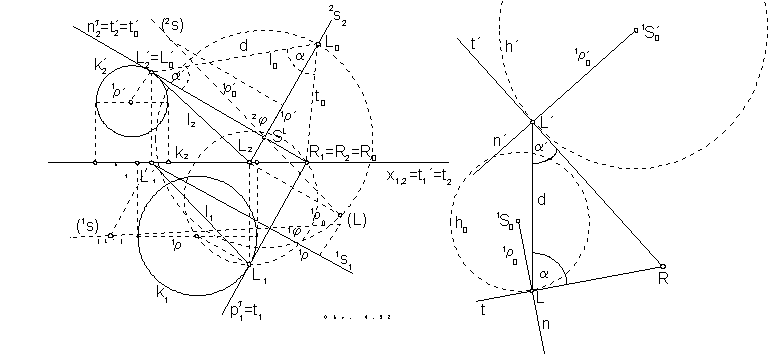
Triangle PSS0 has a right angle in the vertex S - centre of the first curvature in the point P on the curve k located in the plane w. Leg PS of the triangle PSS0 has the lenght equal to the radius of the first curvature 1r of the curve and it forms the angle j with the hypotenuse PS0 from the plane t. Lenght of the hypotenuse is equal to the radius of the first curvature 1r0 in the point P on the curve k0 developed to the plane t.
Points S, S0 are centres of osculating circles h, h0
of curves k, k0 in the common point P,
their radii are the radii of the first cuvatures 1r, 1r0.
t x w=t
![]() t w = j SS0^a
t w = j SS0^a
For j = 0 0 is the curve k developed to its osculating plane in the given point P,
to the plane curve k0 with the same radius of the first curvature 1r = 1r0 .
For j = 900 is the curve k developed to its tangent t located in the tangent plane t
perpendicular to the osculating plane w in the point P with the radius of the first curvature 1r 0 =
![]() .
.
Patch of the developable line surface can be developed to one from its tangent planes t,
which is tangent to the surface in one line
l=1P 2P, 1PÎ1k, 2PÎ2k.
Let the curves 1k, 2k be plane curves located in the osculating planes 1w, 2w.
For the development we must satisfy the following rules:
1. Length of the line segments on the surface lines is preserved invariant d = | 1P 2P |
2. Size of angles of basic curves with the surface lines is preserved invariant
1a = |
![]() 1k l | = |
1k l | = |
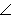 1t l | 2a = |
1t l | 2a = |
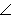 2k l | = |
2k l | = |
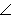 2t l |
2t l |
3. Catalano theorem
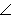 1w t 1r0= 1r cos 1j 2j =
1w t 1r0= 1r cos 1j 2j =
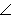 2w t 2r0 = 2r cos 2j
2w t 2r0 = 2r cos 2j 1r 0 , 2r0 are radii of the first curvatures of developed curves 1k0, 2k0 to the tangent plane t in points 1P, 2P.
On normals to these curves (perpendicular to tangents 1t, 2t) there are located centres of curvatures 1S, 2S,
which are centres of osculating circles in the given points, 1h(1S, 1r0), 2h(2S, 2r0).
Developed curve can be in the given point (Fig. 4. 31):
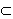 t
t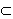 1w and (1t 2P)
1w and (1t 2P)
 t
t 1w and (1t´ 2P´)
1w and (1t´ 2P´)
 t´
t´ 2w and (2t´ 1P´)
2w and (2t´ 1P´)
 t´
t´For the surface of tangents to the space curve the osculating plane w in all points P on the basic curve
coincide with the tangent plane to the surface, w=t, j= 00 and 1r=1r0.
Curve k is developed to the plane curve k0, while the first curvatures of the curves 1k, 1k0 are equal in all points
(surfaces in Fig. 4. 23, 4. 24).
Problem: Find one line l located on the transition surface determined by:
Determine radii of the first curvatures 1r and 1r´ of developed circles to the tangent plane t in the surface line l .
Solution:
 t n
t n.