I. Axioms of Incidence

Axioms in this group describe relation of the superposition of points, lines and planes.

I₁: There exists one and only one line determined by two different points.

I₂: There exist at least two different points located on one line.

I₃: There exists at least one triple of different non-collinear (not located on one line) points.

I₄: There exists one and only one plane determined by three non-collinear points.

I₅: There exists at least one point located in a plane.

I₆: If two different points of a line are located in one plane, then all points of the line are located in this plane.

I₇: There exists a quadruple of non-planar (not located in one plane) points.

I₈: If there exists a common point of two different planes, then there exists a line common to both planes passing through the common point.

 

II. Axioms of Order

Axioms in this group determine relation "to be located inbetween", which will be denoted by d.
The relation that the point C is located inbetween points A and B will be denoted by the symbol d(ACB).

II₁: If the relation d(ACB) is valid, then A, B, C are three different points located on one line and the relation d(BCA) is valid, too.

II₂: There exists at least one point C located on the line p and such, that for an arbitrary pair A, B of different points located on the line p the relation d(ACB) is valid.

II₃: One and only one point from the given triple of different points A, B, C located on one line is located inbetween the two other points, exactly one of relations d(ABC), d(ACB), d(BAC) is valid.

II₄: (Pasch axioma) Let the line p be located in the same plane as the triangle ABC, and it is not passing through any of the triangle vertices, but it intersects one of the triangle sides in the interior point. Then this line intersects at least one more side of the triangle in its interior point.

 

III. Axioms of Congruence

Axioms in this group describe congruence of line segments and congruence of angles.
Congruence of a pair of any other geometric figures is determined by these two basic relations.
One and only one from relations U₁ U₂ , U₁ U₂ is valid for a pair of line segments U₁, U₂.
The same is true for a pair of angles pq, rs,
only one from relations pq rs, pq rs is valid.

III₁: If U is a line segment and a is a half-line with the starting point in the point O, then there exists a point A located on the line a and such, that U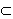OA=a.

III₂: For any line segment AB the following is valid: ABBA.

If U₁ U₂, then U₂ U₁. If U₁ U and U₂ U, then U₁ U₂ .

III₃: If d(ABC), d(A'B' C') are valid, and AB A'B', BC B'C', then AC A'C'.

III₄: Let pq be a given angle, and ( hA ) a given half-plane with the boundary line h, on which a half-line with the starting point O is located. Then there exists the only one half-line with the starting point in the point O located in the half-plane ( hA ) and such, that pq hk.

III₅: For any angle the following is valid: pq qp .
If pq rs is valid, then rs pq is also valid.
If pq rs and rs tv , then pq tv .

III₆: Let two different triangles DABC, DA'B'C' are given, and let the following equalities are valid:
ABA'B', AC A'C', BAC B'A'C'. Then the two triangles are congruent, DABC DA'B'C'.

 

IV. Axioms of Continuity

Axioms of this group determine the terms for measurements of line segments. These measurements must meet certain natural requirements. Length of a line segment must be independent on the place of its realisation and it must be a real positive number. It must be possible to measure the line segments length by parts, while the total length has to be equal to the sum of the partial line segment lengths. There must exist at least one line segment the length of which equals to the unit of measurement. The Archimedean axiom is the result of the experience in a large number of measurements and guarantees the measurability of line segments. An arbitrary line segment can be attached a real number determining its size and denoted as the line segment length. Anyhow, this axiom does not guarantee that an arbitrary real number is the length of at least one line segment. This fact can be ensured by the Cantor axiom.

IV₁: (Archimedean statement)
Let points A, A₁ and B be given on a line and such, that d( A A₁ B ).
Let the sequence of points A₁, A₂, ..., A_k-1, A_k, A_k+1, ...
be determined on the line satisfying the following conditions for any natural number k:
1. d( A_k-1 A_k A_{k +1})
2. A_k A_k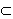+1 AA₁
Then there exists a natural number n, for which d( A A_n B ).

Archimedean axiom ensures the existence of the metric of line segments m(U) - function defined on the set of all line segments, with some specific properties (positive, additive, monotonic), with the set of values in the set of all non-positive real numbers. Any line segment, for which m(J)=1, is denoted as a unit line segment (or a unit) of the metric of line segments m(U).

IV₂: (Cantor statement)
Le a sequence of coherent line segments (A_kB_k 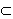A_iB_i, i<k ) be given on a line p. Let for any arbitrary line segment CD there exists a line segment A_kB_k in this sequence, which is its subset, A_kB_k 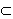CD.
Then a point M exists on the line p, which is the common point of all line segments in the given sequence.

If m is an arbitrary metric of line segments and a is an arbitrary real positive number, then the Cantor axiom ensures the existence of a line segment U, for which m(U) = a.

The set of all congruent, parallel and equally directed (equipolent) line segments in E³ is the vector

a = AB = CD = EF = …, AB CD EF …, AB ­ ­ CD ­­ EF ­­ …

Any line segment of the set is the located vector, B - A = AB = a .
The basic location of a vector is the directed line segment a = OP located to the origin.
Vector a = OP is the position vector of the point P.

The sum or difference of two vectors is a vector, a ± b = c.

Vector is represented by an ordered triple of real numbers, Cartesian coordinates, which are diferrences of the Cartesian coordinates of the directed line segment end points in one arbitrary location of the vector. The vector coordinates are Cartesian coordinates of the end point P in the vector basic location.

a = AB = B - A = [ x_B, y_B, z_B] - [x_A, y_A, z_A ] = [ x_B - x_A, y_B - y_A, z_B - z_A] = [a₁, a₂, a₃ ] = OP

An arbitrary ordered triple of real numbers determines the only one vector in the space E³. 0 = [ 0, 0, 0 ] is a zero vector.
Vectors, for which a = kb, k 0 ( a = kb + lc, k, l 0 ), are linearly dependent - collinear (complanar).

Extended Euclidean Space E³

Any plane a = ap in the space E³ will be extended by the set of ideal points on all lines in the plane B Î p, A Î a, by the ideal line l = AB, and it will become the extended Euclidean plane E².
All planes in the pencil of planes in the space E³ will be extended by the same line. The set of all points extending the space E³ form the plane l at infinity and the resulting space is the extended Euclidean space E³.
Any line in the ideal plane l is the line at infinity l and any point is the point at infinity A, B.
Other points, lines and planes in the space E³ are real figures - P, c, a.
Extended Euclidean space E³ is the model of the projective space.
Any two different planes in E³ are intersecting planes and their common intersection (a pierce line) can be a real line or a line at infinity.
Any two different lines in E³ are intersecting or skew lines, their common intersection is the set of the only one common point ( a pierce point) - real or ideal, or it is an empty set.

Extending geometric figures at infinity "close" lines and planes in the space E³ , and the relation of order in the former Euclidean space is of no sense any more (axioms in the group II.).
Metric of the Euclidean space does not apply to the extending figures at infinity in the space E³. Set of all real points in the space E³ does not change its affinne structure, metric properties valid in the Euclidean space E³.
This fact qualifies the set of all real points in the space E³ as a subset invariant under the metric transformations of the space. All relations and operations defined for figures in the space E³ will be valid also in the space E³, for figures consisting of real points, while in calculations the Cartesian coordinates of points will be used.

Homogeneous system of coordinates in the projective space E³ is the extension of the Cartesian coordinate system in E³ (obr. 1.3).

A set of homogeneous coordinates of the point A = [x_a, y_a, z_a] in the Euclidean space E³ is any ordered quadruple of real numbers ( a₁, a₂, a₃, a₄ ), a₄ 0, for which the following equalities are valid:

The coordinate a₄ is nonzero for any real point in the space E³.
The basic form of the homogeneous coordinates of the finite point A is the ordered quadruple

( x_a, y_a, z_a, 1).

Notion "point at infinity" will be often substituted by notion "direction", parallel lines are in the same direction - they are intersecting in the common point at infinity. It is therefore required, that the point at infinity be represented by a direction vector of any line pointing to the common ideal point. Vectors used in the Euclidean space can be introduced in the same sense also in the space E³. The definiton as a set of all equipolent line segments will determine the homogeneous coordinates of a vector.

An arbitrary location of vector a, directed line segment determined by the end points a = BC, is defined by finite points in the space. Homogeneous coordinates of vector a can be calculated as differences of homogeneous coordinates of the vector end points in the basic form. Homogeneous coordinates of vector representing point at infinity U are homogeneous coordinates of this ideal point. All collinear vectors represent the same point at infinity.

a = C - B = ( x_c, y_c, z_c, 1 ) - ( x_b, y_b, z_b, 1 ) = ( x_c - x_b, y_c - y_b, z_c - z_b, 1 ) = ( x_u, y_u, z_u, 0 )

Any ordered quadruple of real numbers
( x_u, y_u, z_u, 0 ) = k( a₁, a₂, a₃, 0 ), form homogeneous coordinates of an ideal point in the space E³, where numbers a₁, a₂, a₃ are Cartesian coordinates of the point arbitrary representative.