Arithmetic Operations on Complex Numbers

1 Addition and subtraction

Addition of two complex numbers $Complex_Arith_files\Complex_Arith_MathML_0.jpg$ and $Complex_Arith_files\Complex_Arith_MathML_1.jpg$ is carried out thus:

$Complex_Arith_files\Complex_Arith_MathML_2.jpg$ $Complex_Arith_files\Complex_Arith_MathML_3.jpg$ $Complex_Arith_files\Complex_Arith_MathML_4.jpg$ .

The real part of $Complex_Arith_files\Complex_Arith_MathML_5.jpg$ is therefore $Complex_Arith_files\Complex_Arith_MathML_6.jpg$ and the imaginary part is $Complex_Arith_files\Complex_Arith_MathML_7.jpg$ .

In a similar way,

$Complex_Arith_files\Complex_Arith_MathML_8.jpg$ $Complex_Arith_files\Complex_Arith_MathML_9.jpg$ $Complex_Arith_files\Complex_Arith_MathML_10.jpg$ .

The real part of $Complex_Arith_files\Complex_Arith_MathML_11.jpg$ is therefore $Complex_Arith_files\Complex_Arith_MathML_12.jpg$ and the imaginary part $Complex_Arith_files\Complex_Arith_MathML_13.jpg$ .

1.1 Examples

If $Complex_Arith_files\Complex_Arith_MathML_14.jpg$ and $Complex_Arith_files\Complex_Arith_MathML_15.jpg$ what is

i) $Complex_Arith_files\Complex_Arith_MathML_16.jpg$

ii) $Complex_Arith_files\Complex_Arith_MathML_17.jpg$

Solutions:

i) $Complex_Arith_files\Complex_Arith_MathML_18.jpg$ .

ii) $Complex_Arith_files\Complex_Arith_MathML_19.jpg$ .

2 Multiplication

Multiplication of two complex numbers $Complex_Arith_files\Complex_Arith_MathML_20.jpg$ and $Complex_Arith_files\Complex_Arith_MathML_21.jpg$ is carried out thus:

$Complex_Arith_files\Complex_Arith_MathML_22.jpg$ .

The real part of $Complex_Arith_files\Complex_Arith_MathML_23.jpg$ is therefore $Complex_Arith_files\Complex_Arith_MathML_24.jpg$ and the imaginary part is $Complex_Arith_files\Complex_Arith_MathML_25.jpg$ .

2.1 Examples

If $Complex_Arith_files\Complex_Arith_MathML_26.jpg$ and $Complex_Arith_files\Complex_Arith_MathML_27.jpg$ what is

i) $Complex_Arith_files\Complex_Arith_MathML_28.jpg$

ii) $Complex_Arith_files\Complex_Arith_MathML_29.jpg$

Solutions:

$Complex_Arith_files\Complex_Arith_MathML_30.jpg$ $Complex_Arith_files\Complex_Arith_MathML_31.jpg$ .

ii)

$Complex_Arith_files\Complex_Arith_MathML_32.jpg$ .

3 Division

Division is achieved through use of the complex congugate, $Complex_Arith_files\Complex_Arith_MathML_33.jpg$ , i.e. $Complex_Arith_files\Complex_Arith_MathML_34.jpg$ with the sign of the imaginary part reversed. It will be noted that

$Complex_Arith_files\Complex_Arith_MathML_35.jpg$ i.e. a real, positive number.

Division of a complex number $Complex_Arith_files\Complex_Arith_MathML_36.jpg$ by another complex number $Complex_Arith_files\Complex_Arith_MathML_37.jpg$ is facilitated by multiplying both numerator and denominator by $Complex_Arith_files\Complex_Arith_MathML_38.jpg$ giving

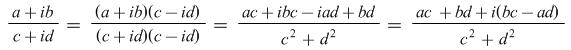$Complex_Arith_files\Complex_Arith_MathML_39.jpg$ .

3.1 Examples

Convert the fraction $Complex_Arith_files\Complex_Arith_MathML_40.jpg$ to the form $Complex_Arith_files\Complex_Arith_MathML_41.jpg$ .

Solution:

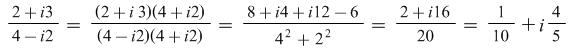$Complex_Arith_files\Complex_Arith_MathML_42.jpg$ .

4 Powers of complex numbers

Powers of pure imaginary numbers of the form $Complex_Arith_files\Complex_Arith_MathML_43.jpg$ are found by calculating $Complex_Arith_files\Complex_Arith_MathML_44.jpg$ and $Complex_Arith_files\Complex_Arith_MathML_45.jpg$ separately and multiplying the two results together.

4.1 Example

Calculate $Complex_Arith_files\Complex_Arith_MathML_46.jpg$ where $Complex_Arith_files\Complex_Arith_MathML_47.jpg$ .

Solution:

$Complex_Arith_files\Complex_Arith_MathML_48.jpg$ . $Complex_Arith_files\Complex_Arith_MathML_49.jpg$ .

$Complex_Arith_files\Complex_Arith_MathML_50.jpg$ .

Therefore,

$Complex_Arith_files\Complex_Arith_MathML_51.jpg$ .

Powers of complex numbers of the form $Complex_Arith_files\Complex_Arith_MathML_52.jpg$ can be calculated by expanding $Complex_Arith_files\Complex_Arith_MathML_53.jpg$ . However, for large values of $Complex_Arith_files\Complex_Arith_MathML_54.jpg$ this calculation may be tedious, or, indeed, impractical.

An easier method is to use de Moivre's formula

$Complex_Arith_files\Complex_Arith_MathML_55.jpg$ .

4.2 Example

Calculate $Complex_Arith_files\Complex_Arith_MathML_56.jpg$ where $Complex_Arith_files\Complex_Arith_MathML_57.jpg$ .

Solution:

The modulus of $Complex_Arith_files\Complex_Arith_MathML_58.jpg$ is found by $Complex_Arith_files\Complex_Arith_MathML_59.jpg$ .

The argument of $Complex_Arith_files\Complex_Arith_MathML_60.jpg$ is found by $Complex_Arith_files\Complex_Arith_MathML_61.jpg$ (noting that $Complex_Arith_files\Complex_Arith_MathML_62.jpg$ is in the first quadrant).

$Complex_Arith_files\Complex_Arith_MathML_63.jpg$ can therefore be re-written as

$Complex_Arith_files\Complex_Arith_MathML_64.jpg$

Using de Moivre's formula,

$Complex_Arith_files\Complex_Arith_MathML_65.jpg$ $Complex_Arith_files\Complex_Arith_MathML_66.jpg$ .

5 Roots of complex numbers

Roots of complex numbers are also found using de Moivre's formula, this time in the form

$Complex_Arith_files\Complex_Arith_MathML_67.jpg$ .

When dealing with real numbers, we are used to there being a maximum of two solutions when calculating roots i.e. two solutions, $Complex_Arith_files\Complex_Arith_MathML_68.jpg$ , for even roots, and one solution, $Complex_Arith_files\Complex_Arith_MathML_69.jpg$ , for odd roots. However, when calculating complex roots, there are $Complex_Arith_files\Complex_Arith_MathML_70.jpg$ different solutions for $Complex_Arith_files\Complex_Arith_MathML_71.jpg$ roots.

5.1 Examples

i) Find the complex 4th roots of $Complex_Arith_files\Complex_Arith_MathML_72.jpg$ .

ii) Find the complex 3rd (cube) roots of $Complex_Arith_files\Complex_Arith_MathML_73.jpg$ .

i) Solution:

The modulus of $Complex_Arith_files\Complex_Arith_MathML_74.jpg$ is clearly given by $Complex_Arith_files\Complex_Arith_MathML_75.jpg$ . The argument of $Complex_Arith_files\Complex_Arith_MathML_76.jpg$ is zero, since $Complex_Arith_files\Complex_Arith_MathML_77.jpg$ lies on the positive real axis. The complex 4th roots of $Complex_Arith_files\Complex_Arith_MathML_78.jpg$ are therefore given by

$Complex_Arith_files\Complex_Arith_MathML_79.jpg$

i.e. the roots are $Complex_Arith_files\Complex_Arith_MathML_80.jpg$ . The results can easily be verified by calculation.

ii) Solution: The modulus of $Complex_Arith_files\Complex_Arith_MathML_81.jpg$ is given by $Complex_Arith_files\Complex_Arith_MathML_82.jpg$ .

The argument of $Complex_Arith_files\Complex_Arith_MathML_83.jpg$ is $Complex_Arith_files\Complex_Arith_MathML_84.jpg$ , since $Complex_Arith_files\Complex_Arith_MathML_85.jpg$ lies in the 2nd quadrant of the Argand diagram.

The complex cube roots of $Complex_Arith_files\Complex_Arith_MathML_86.jpg$ are therefore given by

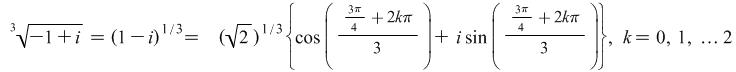
$Complex_Arith_files\Complex_Arith_MathML_87.jpg$

$Complex_Arith_files\Complex_Arith_MathML_88.jpg$

$Complex_Arith_files\Complex_Arith_MathML_89.jpg$